Let us walk and confer together, my son, for I am eager to learn your impressions of the new school.
There is much to tell, Father. Beginning students at Semicircle are not permitted to speak aloud for the first three years. We are instructed to listen carefully but forbidden to make written notes. The Teacher insists that we internalize the lessons rather than routinely memorize content.
The advanced students are able discuss the lessons with Pythagoras, is that not the case?
It is so, but for now we are silenced.
What manner of instruction has transpired?
There are many things and sundry. Today we observed the initiates as they explored relationships between the lengths of sides in a right triangle.
I am but a tiller of the soil, my son. Please elaborate.
A thousand pardons, Father. I have been cautioned that on occasion my excitement with discovery exceeds my reasoning and proper manners. If I may continue, these corners of your fields are formed in a manner that The Teacher classifies as ‘right’ angles, each measuring 90 degrees. A right triangle contains one of these right angles. The longest side of a right triangle is called ‘hypotenuse’ and is always opposite the right angle. The area of a square constructed on that side of the triangle will be equal to the sum of areas constructed on squares of the other two sides.
Yes, I understand that geometry is at the foundation of ownership. It is required that we respect boundaries and the adjacent property of a neighbors’ field.
All our lessons at Semicircle include reference to the extended application of concepts. To verify his teaching The Teacher drew a diagram that placed four congruent right triangles defining with their right angles four corners of a large square. I can replicate it here in the sand.
I can see that the remaining area is a smaller square whose sides are delineated by the length of the hypotenuse.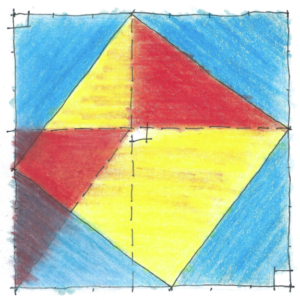
Exactly, but when I draw a second diagram thus, with the same four triangles grouped in pairs that define two intersecting rectangles, the remaining area is, by contrast, two smaller squares. It follows that the sum of their areas is the same as the area of the remaining single square in the first diagram. Have I demonstrated that to you, Father?
The hour is late, Alex. Tomorrow we will speak more of these things.
Harpeth Rivers is a writer, musician and happy homeowner still living and working in New Mexico. His latest book, Proof, an illustrated fable, is available at Amazon.
